CONFIDENCE function

Returns the confidence interval for a population mean with a normal distribution. The confidence interval is a range on either side of a sample mean. For example, if you order a product through the mail, you can determine, with a particular level of confidence, the earliest and latest the product will arrive.

Syntax

CONFIDENCE(alpha,standard_dev,size)

Alpha     is the significance level used to compute the confidence level. The confidence level equals 100*(1 - alpha)%, or in other words, an alpha of 0.05 indicates a 95 percent confidence level.

Standard_dev     is the population standard deviation for the data range and is assumed to be known.

Size     is the sample size.

Remarks

• If any argument is nonnumeric, CONFIDENCE returns the #VALUE! error value.

• If alpha ≤ 0 or alpha ≥ 1, CONFIDENCE returns the #NUM! error value.

• If standard_dev ≤ 0, CONFIDENCE returns the #NUM! error value.

• If size is not an integer, it is truncated.

• If size < 1, CONFIDENCE returns the #NUM! error value.

• If we assume alpha equals 0.05, we need to calculate the area under the standard normal curve that equals (1 - alpha), or 95 percent. This value is ± 1.96. The confidence interval is therefore:

  

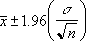
Example

Suppose we observe that, in our sample of 50 commuters, the average length of travel to work is 30 minutes with a population standard deviation of 2.5. We can be 95 percent confident that the population mean is in the interval:

Alpha	StdDev	Size	Formula	Description (Result)
0.05	.5	50	=CONFIDENCE([Alpha],[StdDev],[Size])	Confidence interval for a population mean. In other words, the average length of travel to work equals 30 ± 0.692951 minutes, or 29.3 to 30.7 minutes. (0.692951)